Featured publication
V. Sharoglazova, M. Puplauskis, C. Mattschas, C. Toebes, and J. Klaers, “Energy-speed relationship of quantum particles challenges Bohmian mechanics”, Nature 643, 67 (2025). link
Quantum mechanics is compatible with a range of fundamentally different models (often referred to as interpretations), each offering a distinct account of what constitutes physical reality. For example, while the theory is commonly associated with intrinsic randomness, indeterminism is not an established empirical fact. Deterministic models of quantum phenomena exist—most prominently Bohmian mechanics, which posits definite particle trajectories guided by the quantum wave function via the so-called guiding equation. It is often argued that Bohmian mechanics is empirically equivalent to standard quantum mechanics. This equivalence is typically understood in terms of the particle density: if the initial conditions—i.e., the starting points of the Bohmian trajectories—follow the Born rule, then the predicted particle density, averaged over many trajectories, matches that of standard quantum theory. It is further argued that, since every observable can, in principle, be reduced to a position measurement—for example, spin can be inferred from particle trajectories in a Stern–Gerlach experiment—Bohmian mechanics is expected to reproduce all standard quantum measurement predictions.
However, empirical adequacy is only a necessary condition for the validity of a physical theory. A theory must also be internally consistent. To illustrate this important point, consider a system designed to measure particle velocity by mapping it onto a position measurement—for example, using a carefully engineered potential landscape. Solving the Schrödinger equation for such a system yields a specific particle density from which a velocity can be inferred. Since standard quantum mechanics and Bohmian mechanics agree on the density, they will also agree on the inferred velocity.
But in Bohmian mechanics, particle velocities are not merely inferred—they are explicitly defined by the guiding equation. Importantly, there is no fundamental reason why the velocity obtained from a position-based measurement must coincide with the one prescribed by the guiding equation. It is well known that the choice of guiding equation in Bohmian mechanics is not unique. If an experiment reveals a mismatch between the measured and the prescribed velocity, it would indicate an internal inconsistency: the theory assigns a velocity that differs from what a suitable measurement device records. Such a contradiction undermines the internal consistency of the theory—even if it remains empirically adequate in terms of the particle density. This is precisely the logic we pursued in a recent experiment.
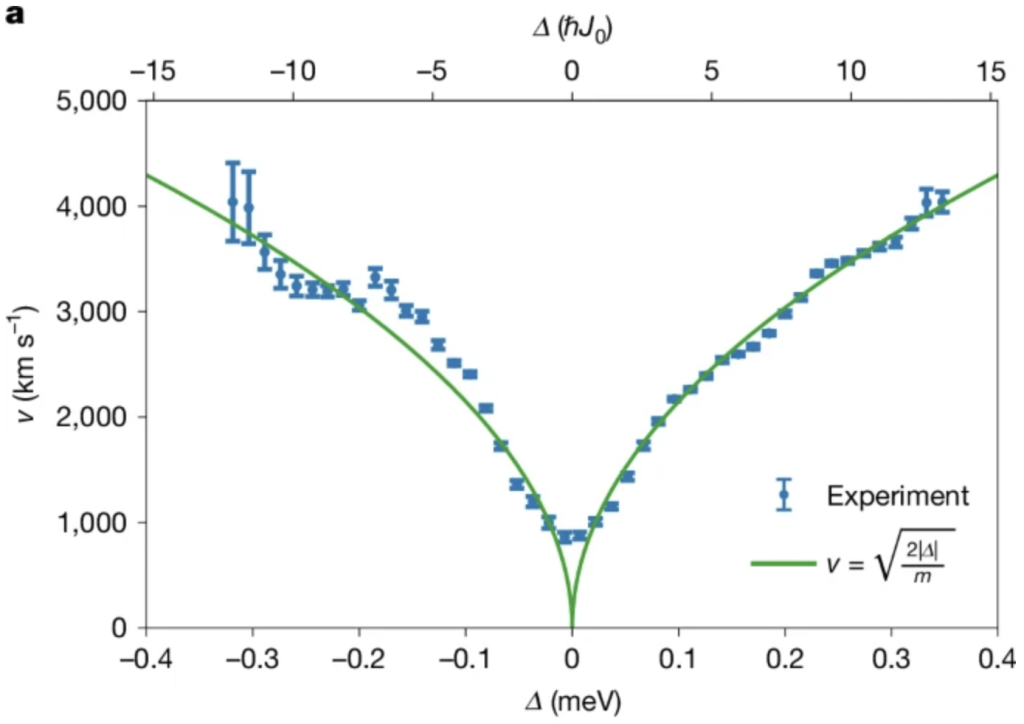
We have recently investigated the particle motion associated with evanescent wave functions, which appear when particles are reflected at a potential step. To do this, we confine the particles to one-dimensional waveguides and couple two such waveguides in a controlled manner by placing them parallel to each other at a close distance. The coupling between the waveguides provides a temporal reference for the particles’ motion. By comparing the motion of particles within a waveguide to the tunneling-induced hopping between the waveguides, we can draw conclusions about how fast the particles move.
The outcome of our experiments is that particles move where they are not expected to move. Contrary to the predictions of the Bohmian guiding equation, which for evanescent wavefunctions suggest that particles are at rest, our experimental results indicate that particles move with a well-defined speed. The measured speed supports the core idea behind de Broglie’s relation: that motion and wavelength are fundamentally linked—albeit with a twist. For evanescent wave functions, we find that a de Broglie relation of the form λ=ℏ/mv holds, where λ is the decay length and v represents the non-directional particle speed that we measure in our experiment. Thus, our results suggest that phase gradients and amplitude gradients play complementary roles in indicating motion within a quantum mechanical wave function. This stands in contrast to the guiding equation in Bohmian mechanics, which attributes motion solely to phase gradients. In other words, our findings call into question whether the ontology implied by Bohmian trajectories is realized in nature.
Featured publication
J. Klaers, V. Sharoglazova and C. Toebes, “Particle motion associated with wave-function density gradients”, Physical Review A 107, 052201 (2023). link
In our everyday life, it seems obvious that physical objects have a position and a velocity. This intuitive understanding is, however, seriously challenged by quantum mechanics. Quantum mechanics knows a variety of velocity definitions such as group velocity, phase velocity, velocities derived from the momentum operator or from the probability flow. However, their physical meaning is not always obvious and may not correspond to our classical understanding of motion.
Assigning a velocity to a wavefunction is possible in some cases, such as with Gaussian wave packets, and may even fit our classical intuition of motion quite well. However, the situation becomes much less obvious when this wave packet meets a reflective potential step, so that the oscillating behavior of the wavefunction turns into an exponential decay. Is it still possible to assign a physically meaningful velocity to the exponentially decaying part of the wavefunction? This question was the starting point for our work.
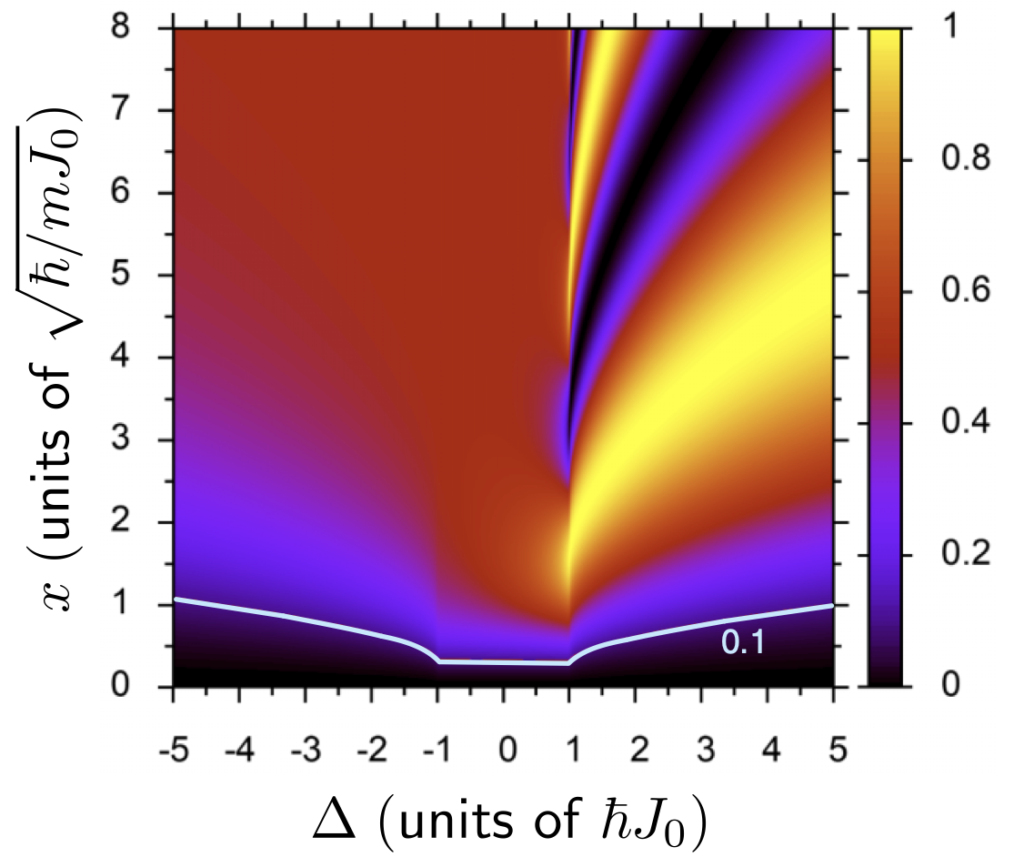
To investigate this question, we have adapted an approach known from tunneling time research, the so-called Larmor clock, for our purposes. This approach basically means adding additional degrees of freedom to the system, the change of which can be understood as a measurement of time. More specifically, we study the quantum mechanical motion of massive particles in a system of two coupled waveguide potentials, where the population transfer between the waveguides effectively acts as a clock and allows particle velocities to be determined. The application of this scheme to tunneling phenomena at a reflective step potential leads to the main results of our work, namely an energy-velocity relationship for classically forbidden motion.